English
Español
Deutsch
Français
日本語
1. Heat conduction-optimize the heat spreading resistance of the radiator
When a heat sink is attached to an electronic component, the heat transfer from the inside of the device to the radiator and the heat transfer inside the radiator belong to heat conduction. Heat conduction in classical heat transfer can be described by the Fourier heat conduction formula:
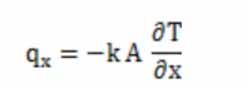
It can be seen from the above formula that thermal conductivity and thermal cross-sectional area are two key variables that affect heat transfer efficiency in heat conduction.
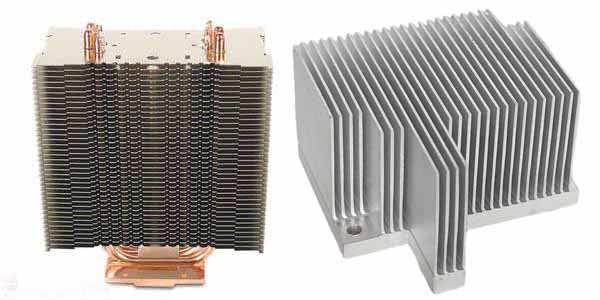
Among the common metals, aluminum alloys and copper alloys have better thermal conductivity and comprehensive economic performance. Therefore, the common heat sink are mainly aluminum extrusion heat sink and copper heat sink.
2. Convective heat transfer-enhancing the efficiency of convective heat transfer
After the heat of the components is transferred to the radiator through heat conduction, it is necessary to transfer the heat radiator to the environment through convection and radiation heat exchange to complete the heat dissipation. The heat exchange method between the radiator fins and the surrounding air is convective heat exchange. Let's first look at Newton's law of cooling used to describe convective heat transfer:
Obviously, by increasing the convective heat transfer area, heat transfer can be directly enhanced. However, increasing the heat transfer area usually means that the radiator has to be larger in size, which in turn causes the overall size of the product to become larger. This is not in line with the trend of increasingly compact electronic products. In addition, in most cases, increasing the radiator also means increasing the cost of heat dissipation. When the space is given, increasing the heat dissipation area must also consider the system wind resistance, because the fine radiator increases the heat dissipation area while increasing the wind resistance, which affects the internal air flow, thereby reducing the convective heat transfer coefficient. A conventional phenomenon is sufficient to explain the relationship between fin density and wind resistance: the radiator fin density of forced air-cooled products is usually higher than that of natural heat dissipation products.
In addition to simply changing the tooth spacing of the radiator to obtain a higher convective heat transfer coefficient, the broken teeth, helical teeth, and blooming teeth of the radiator are all trade-offs between the heat dissipation area and the convective heat transfer coefficient. Optimize the heat dissipation effect by reducing the wind resistance and the effect of inhaling cold air in the gap.
In system-level products, the optimization of the combination of heat sink design, fan selection and air duct design is quite complicated. When there are multiple heating points, multiple heat sinks, and multiple fans, the components need to cooperate with each other to effectively use the system air volume, weaken the cascading effect between hot spots, and achieve the optimal design combination.
+86-18902844286
E-mail
